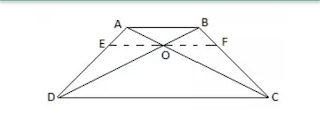
Sum no. 10 Exercise 6.2 class 10th

Sum No.10:- T he diagonals of a quadrilateral ABCD intersect each other at a point O such that AO/BO=CO/DO. show that ABCD is a trapezium. Solution:- Given :- ABCD is a quadrilateral, whose diagonals AC and BD intesect each other at O. such that AO/BO=CO/DO i.e., AO/CO=BO/DO......(1) To prove :- ABCD is a trapezium. Construction :- from O,draw OE∥AB. Proof :- In △ADB, we have, OE∥AB (construction) ∴ by BPT, we have AE/ED=BO/DO.....(2) from (1) and (2),we have AO/CO=AE/ED thus in △ADC,E and O are points dividing the sides AD and AC in the same ratio. ∴ by converse of BPT, we have {OE∥CD but OE∥AB by construction} hence OE∥CD∥AB ⇒ AB∥CD ∴ Quadrilatral ABCD is at Trapezium.