class 10th Exercise 6.2, sum no.9

                                             EXERCISE 6.2
SUM NO.9
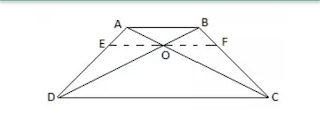
ABCD is trapezium in which AB∥DC and its diagonals intersect each other at the point O.show that AO/BO=CO/DO.

 Solution:- 
Given:-In trapezium ABCD,AB∥DC, diagonal AC and BD intersect at o.

Construction:- Draw OE∥DC, intersecting AD at E.
Proof:- in △ ADC, OE∥DC(by construction)
   ∴ AE/ED=AO/OC......(1)

         (by Basic proportionality theorem)
Again,in △ABD,OE∥AB
AE/ED=BO/OD.....(2)
[∵ OE∥ DC (by construction) and DC∥AB (given) also by by Basic proportionality theorem)
 From (1) and (2) we have:
AO/CO=BO/OD

 AO/BO=CO/DO

 Hence proved

Comments

Post a Comment

Popular posts from this blog

Basic proportionality Theorem or Thales Theorem class 10th

Image
Imp points or results used in  Basic proportionality theorem or Thales theorem:- (i) Area of Triangle = 1/2 base* height Area of Triangle= 1/2 base*height                     =1/2 BC*AD (ii) Area of obtuse triangle =  In this figure CM is height of the triangle and AB is the base Area of triangle = 1/2 base* height                    =1/2 AB* CM (iii) Two triangles on the same base (or equal bases) and between the same parallels are equal in areas. (class 9th) There are two triangle in this figure △CDA and △CDB so,  ar(CDA)= ar(CDB) Theorem 6.1 if a line is drawn parallel to one side of triangle to intersect the other two sides in distinct points, the other two sides are divided in the same ratio .   Proof  Given:-    triangle ABC in which a line l parallel to side BC intersects other two sides AB and AC at D and E respec...
Read more

EXERCISE 6.2 sum no.4 (class 10th )

Image
              EXERCISE 6.2 SUM 4.    In a figure, DE∥AC and DF∥ AE, prove that BE/FE=BE/EC. Solution:-  Given:-  DE∥AC  and DF∥ AE To Prove :-  BE/FE=BE/EC Proof:- In △ABC  DE∥AC  Because ( if a line is drawn parallel to one side of triangle to intersect the other two sides in distinct points, the other two sides are divided in the same ratio .) BE/EC=BD/DA        .......(1) and, In △AEB DF∥ AE Because  ( if a line is drawn parallel to one side of triangle to intersect the other two sides in distinct points, the other two sides are divided in the same ratio .) BF/FE= BD/DA            ......(2)     From (1) and (2)   BE/FE=BE/EC Hence proved 
Read more

Sum no. 10 Exercise 6.2 class 10th

Image
Sum No.10:- T he diagonals of  a quadrilateral ABCD intersect each other at a point O such that AO/BO=CO/DO. show that ABCD is a trapezium. Solution:- Given :- ABCD is a quadrilateral, whose diagonals AC and BD intesect each other at O. such that AO/BO=CO/DO i.e., AO/CO=BO/DO......(1) To prove :- ABCD is a trapezium. Construction :- from O,draw OE∥AB. Proof :- In △ADB, we have,              OE∥AB (construction) ∴ by BPT, we have  AE/ED=BO/DO.....(2) from (1) and (2),we have AO/CO=AE/ED thus in △ADC,E and O are points dividing the sides AD and AC in the same ratio. ∴ by converse of BPT, we have {OE∥CD but OE∥AB by construction} hence OE∥CD∥AB ⇒ AB∥CD ∴ Quadrilatral ABCD is at Trapezium.
Read more